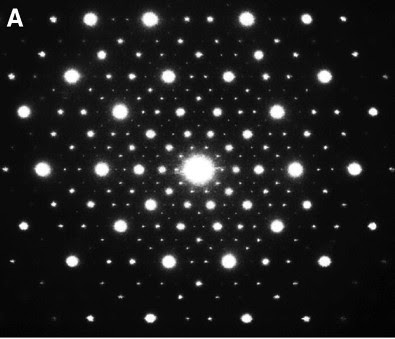
 Explorations of five-fold symmetry on the plane go back to Persian artists of the twelfth century. I became aware of this when I read Critchow ("Islamic Patterns" 1976), which presents "demonstrations" of "important shapes that enable the five-fold symmetry to harmonize with the necessary repeats of a two-dimensional surface." The matter of Islamic quasicrystalline tilings was taken up in detail by Lu and Steinhardt (who are also authors on this new paper) in 2007 ("Decagonal and Quasi-Crystalline Tilings in Medieval Islamic Architecture"). However, it was only in the 1970s that Penrose tilings, a periodic but infinite tilings with fivefold symmetry, were described.
Explorations of five-fold symmetry on the plane go back to Persian artists of the twelfth century. I became aware of this when I read Critchow ("Islamic Patterns" 1976), which presents "demonstrations" of "important shapes that enable the five-fold symmetry to harmonize with the necessary repeats of a two-dimensional surface." The matter of Islamic quasicrystalline tilings was taken up in detail by Lu and Steinhardt (who are also authors on this new paper) in 2007 ("Decagonal and Quasi-Crystalline Tilings in Medieval Islamic Architecture"). However, it was only in the 1970s that Penrose tilings, a periodic but infinite tilings with fivefold symmetry, were described. This is a Penrose tiling showing five-fold symmetry.
This is a Penrose tiling showing five-fold symmetry.More recently, the production of quasicrystals in the laboratory has become something of a cottage industry, but only now have naturally occurring quasicrystals been found.
Most of what I might say is said better in article, so I encourage interested readers to read the original.

